

Answer: E
Solution:
On a cube, there are 12 edges, 8 corners, and 6 faces. Adding them up gets 12+8+6=26.
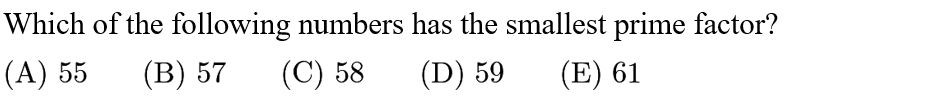
Answer: C
Solution:
The smallest prime factor is 2, and since 58 is the only multiple of 2, the answer is 58.
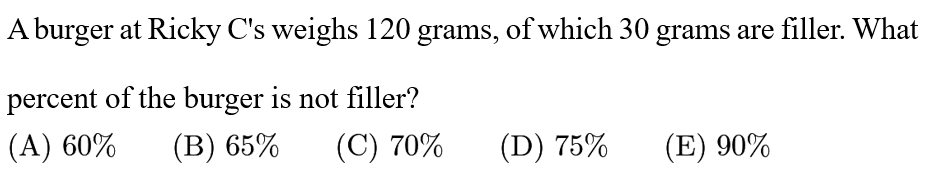
Answer: D
Solution:
There are 30 grams of filler, so there are 120-30=90 grams that aren't filler. 90/120.

Answer: C
Solution:
Setting up an equation, we have a+b=7 children and 3a+2b=19. Solving for the variables, we get a=5 tricycles.
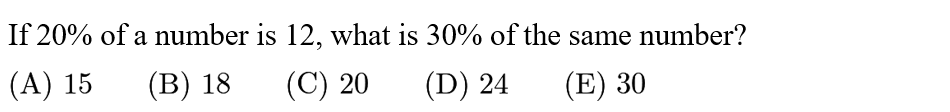
Answer: B
Solution:
Let n=the number, 20%*n=12, so n=60; then 60*30%=18.
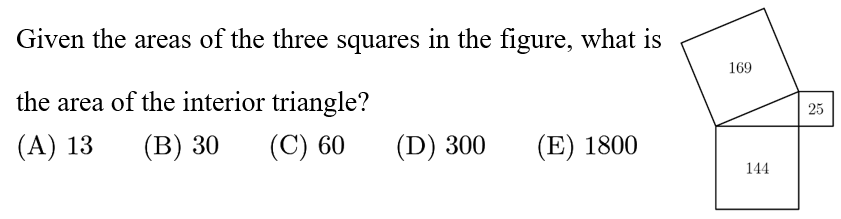
Answer: B
Solution:
The sides of the squares are 5, 12, and 13 for the square with area 25, 144, and169, respectively. The legs of the interior triangle are 5 and 12, so the area is 1/2*12*5=30.

Answer: A
Solution:
The total point difference between Blake’s and Jenny’s scores is 10-10+20+20=40, the average of it is 40/4=10.

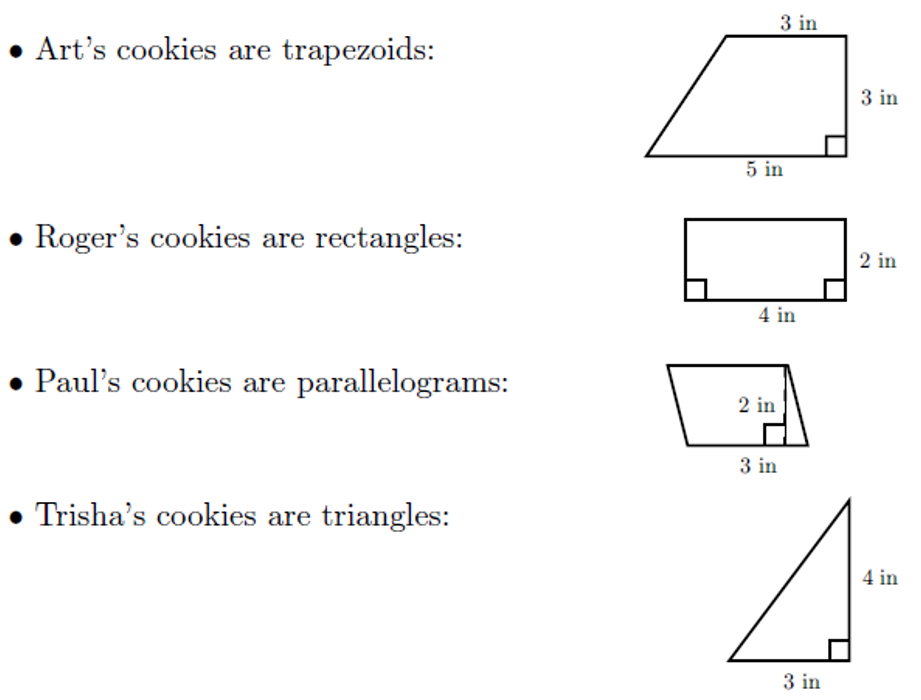
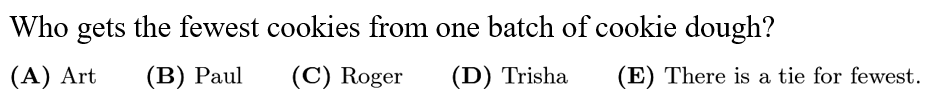
Answer: A
Solution:
The Area of Art’s : 1/2*(3+5)*3=12; The area of Roger’s: 2*4=8; The area of Paul’s : 3*2=6; The area of Trisha’s : 1/2 * 3*4=6;
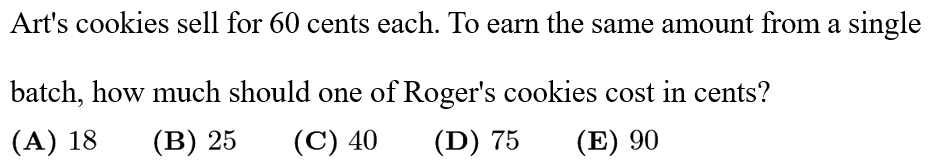
Answer: C
Solution:
The area of one of Art’s cookies is 1/2*(3+5)*3=12; As he has 12 cookies in a batch, the amount of dough each person used is 12*12=144. Roger’s cookies have an area of 144/((2*4)=18 cookies in a batch. In total, the amount of money Art will earn is 12*60=720. Thus, the amount Roger would need to charge per cookie is 720/18=40.
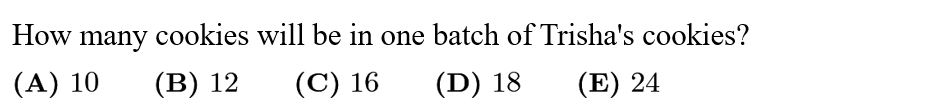
Answer: E
Solution:
Art’s cookies have areas of 1/2*(3+5)*3=12. There are 12 cookies in one of Art’s batches so everyone used 12*12=144 of dough. Trisha’s cookies have an area of 3*4/2=6, so she has 144/6=24 cookies per batch.
Answer: B
Solution:
On Friday, the shoes would cost 40*1.1=44 dollars. Then on Monday, the shoes would cost 44*(1-10%)=44*0.9=39.6 .
Answer: E
Solution:
All the possibilities where 6 is on any of the five sides is always divisible by six, and 1*2*3*4*5 is divisible by 6 since 2*3=6. So, the answer is E because the outcome is always divisible by 6.
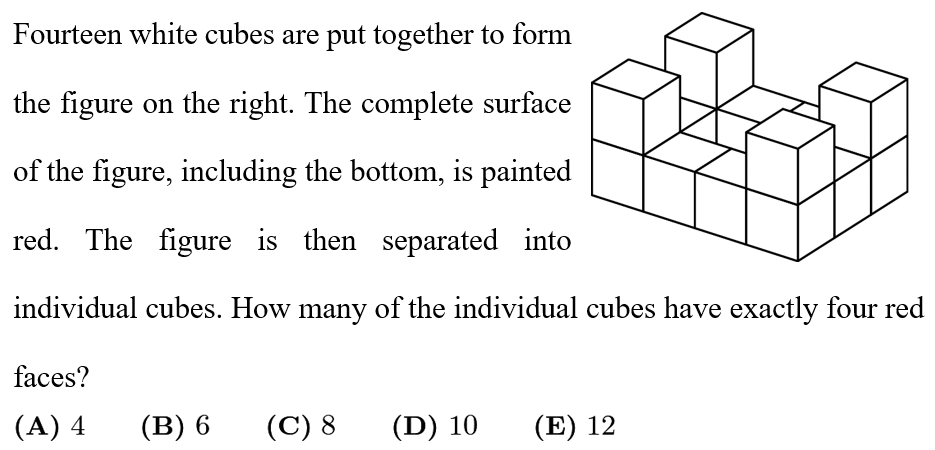
Answer: B
Solution:
This is the number cubes that are adjacent to another cube on two sides. The bottom corner cubes are connected on three sides, and the top corner cubes are connected on one. The number we are looking for is the number of middle cubes, which is 6.

Answer: D
Solution:
Since both T's are 7, then O has to equal 4, because 7 + 7 = 14. Then, F has to equal 1. To get R, we do 4 + 4 (since O = 4) to get R = 8. The value for W then has to be a number less than 5, otherwise it will change the value of O, and can't be a number that has already been used, like 4 or 1. The only other possibilities are 2 and 3. 2 doesn't work because it makes U = 4, which is what O already equals. So, the only possible value of W is 3.
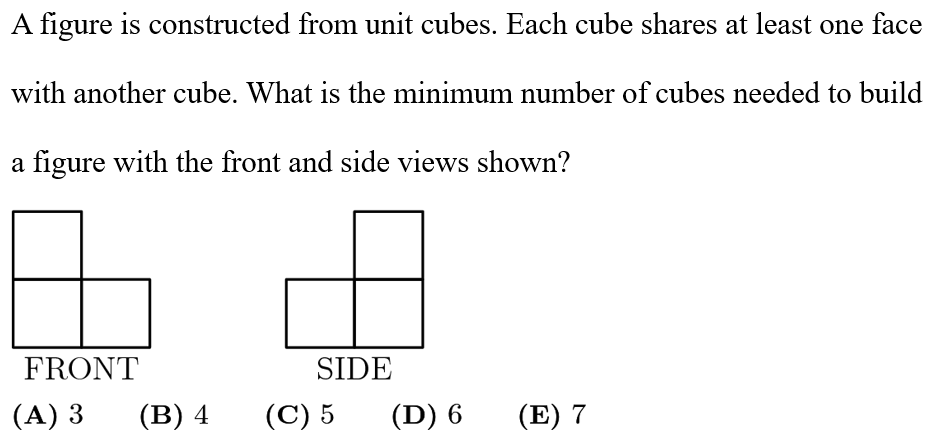
Answer: B
Solution:
In order to minimize the amount of cubes needed, we must match up as many squares of our given figures with each other to make different sides of the same cube. One example of the Solution with 4 cubes. Notice the corner cube cannot be removed for a figure of 3 cubes because each face of a cube must be touching another face.

Answer: D
Solution:
There are only 2 people who can go in the driver's seat--Bonnie and Carlo. Any of the 3 remaining people can go in the front passenger seat. There are 2 people who can go in the first back passenger seat, and the remaining person must go in the last seat. Thus, there are 2*3*2=12 ways.

Answer: E
Solution:
If you look for anybody who has brown eyes or blonde hair, you find that Nadeen, Austin, and Sue are Jim's possible siblings. However, the children have at least one common characteristics. Since Austin and Sue both have blonde hair, Nadeen is ruled out and therefore Austin and Sue are his siblings. You can also see that in the hair color column, there are three black haired people and three blond haired people. Since Jim has blond hair, all his siblings must be the other two with blond hair.
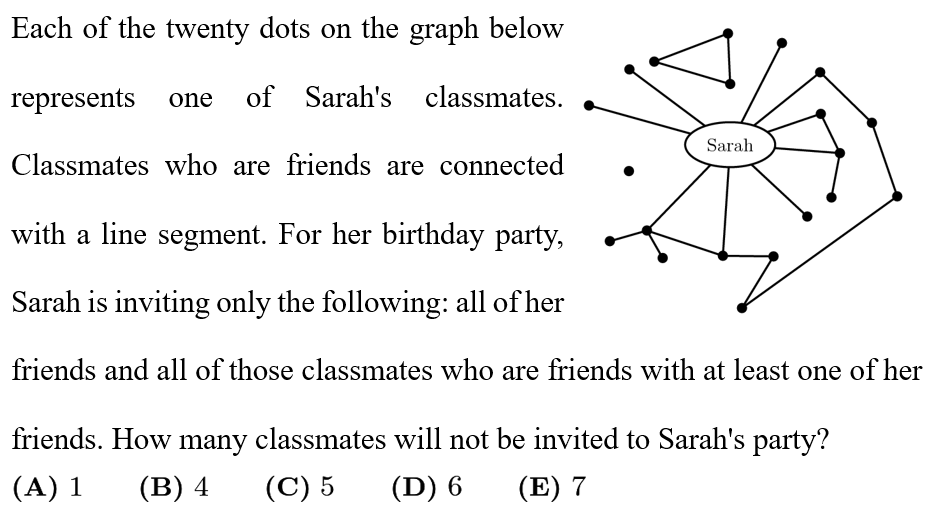
Answer: D
Solution:
There are 3 people who are friends with only each other who won't be invited, plus 1 person who has no friends, and 2 people who are friends of friends of friends who won't be invited. So the answer is 6.

Answer: C
Solution:
The LCM of 15, 20, and 25 is 300. The number of multiples between 1000 and 2000 is 3.

Answer: D
Solution:
By 4:20, the hour hand would have moved 1/3 way from 4 to 5 since 20/60 is reducible to 1/3. . One third of the way from 4 to 5 is one third of 30 degrees, which is 10 degrees past the 4.
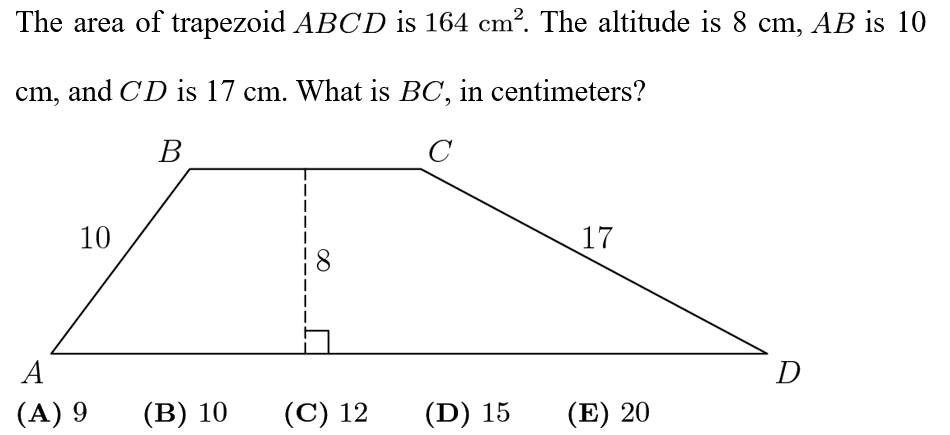
Answer: B
Solution:
Using the formula for the area of a trapezoid, we have 164=1/2*(BC+AD)*8; thus BC+AD=41. Drop perpendiculars from B to AD and from C to AD and let them hit AD at E and F respectively. Note that each of these perpendiculars has length8. From the Pythagorean Theorem, AE=6 and DF=15. BC+(AE+EF+FD)=BC+6+EF+15=BC+6+BC+15=2BC+21. Since BC+AD=41, So BC=10.
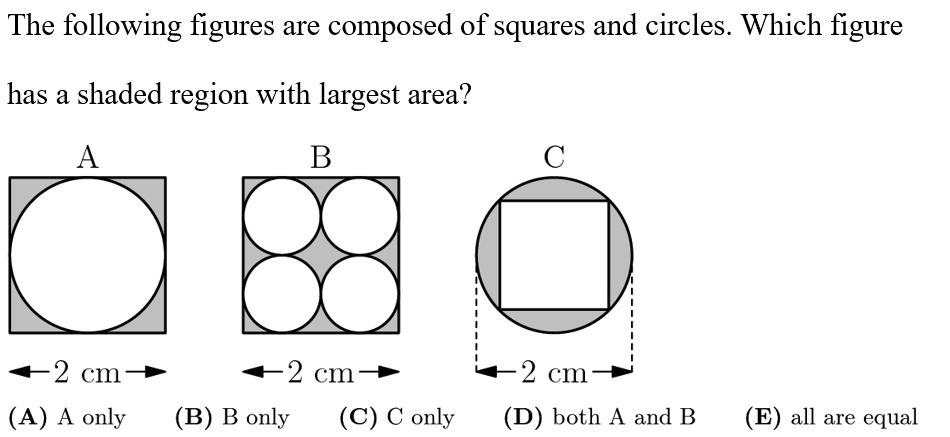
Answer: B
Solution:
Figure A: 2*2-Pi*1*1=4-Pi; Figure B: 2*2-4*(Pi*0.5*0.5)=4-Pi; Figure C: the area of the square is 2*2/2=2; the area of the shaded is Pi*1*1-2=Pi-2; Thus the answer is C.
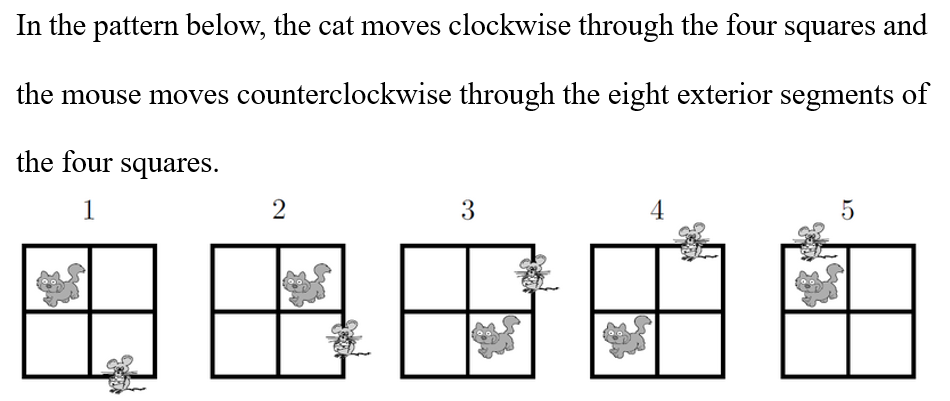
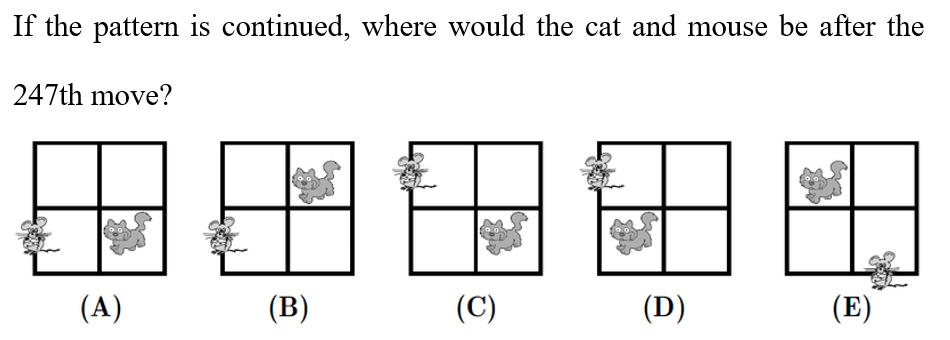
Answer: A
Solution:
The cat has four possible configurations which are repeated every four moves. 247 has a remainder of 3 when divided by 4. This corresponds to the position the cat has after the 3rd move, which is the bottom right corner.
Similarly, the mouse has eight possible configurations that repeat every eight moves. 247 has a remainder of 7 when divided by 8. This corresponds to the position the rat has after the 7th move, which can easily be found by writing two more steps to be the bottom edge on the left side of the grid
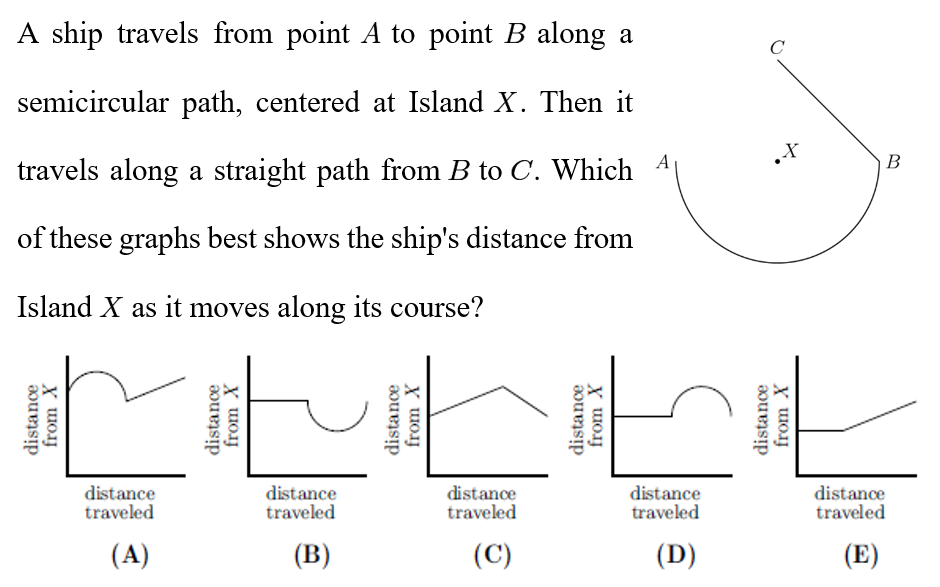
Answer: B
Solution:
The distance from x to any point on the semicircle will always be constant. On the graph, this will represent a straight line. The distance between x and line BC will not be constant though. Since the point on line BC and the perpendicular bisector from vertex X is the shortest distance between X and BC and it less than the radius. Using the information found, the answer choice that fits them all is B.
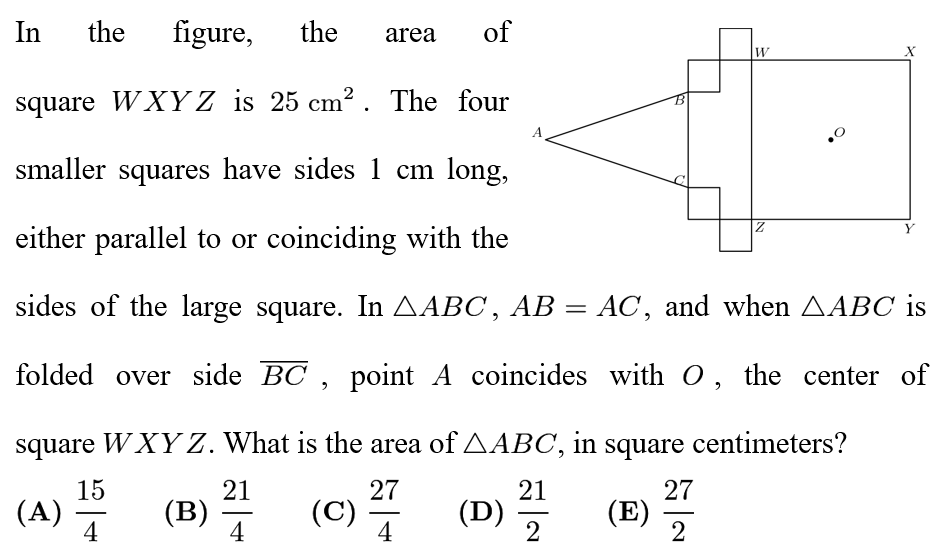
Answer: C
Solution:
The side lengths of square WXYZ must be 5 cm, since the area is 25. First, you should determine the height of triangle ABC. The distance from O to line WZ must be 2.5 cm. The distance from line WZ to line BC must be 2, since the side lengths of the small squares are 1. So, the height of triangle ABC must be 4.5, which is 2.5 + 2. The length of BC can be determined by subtracting 2 from 5, since the length of WZ is 5, and the two squares in the corners give us 2 together. This gives us the base for triangle ABC, which is 3. Then, we multiply 4.5 by 3 and divide by 2, to get an answer of 27/4.
AMC培训、答疑,请联系微信 / 电话:136 1118 1627
艾蕾特教育 @Elite Edu, 在这里,